ASTR330 Homework #2
Homework hint: All problems in the text have answers at the back of
the book! Some problems require just a written response, while
others ask you to calculate something. Please write up all answers
clearly, completely, and as succinctly as possible. You can work with
others, but your final answers must be written up on your own.
Chapter 3
Question 1. Find equation 3.2 for the terminal fall
speed of volcanic material in an atmosphere. Consider the case of 1-cm
diameter piece of volcanic ash falling through Earth's atmosphere.
a) All else being equal, how much faster or slower would material fall:
i) on Earth if it is 4cm in diameter?
ii) on a planet with 9 times weaker gravity?
iii) on Venus with nearly the same gravity, but a 90 times denser atmosphere?
iv) On Mars with 3 times weaker gravity and an atmosphere 200 times
less dense?
You can refer back to
the Working with
Equations program to practice this type of problem.
b) Can you explain why terminal velocity for a skydiver is smaller
(120 mph) when she is in the prone position (looking downward)
than when she is in a head-first diving position (180mph)?
Chapter 4
Question 2. These next questions will all use the Solar
System Collisions website at
http://janus.astro.umd.edu/astro/impact/
a) Investigate collisions with the planets Mars, Venus, and
Earth. Determine the maximum-sized rocky object that is destroyed in the
planet's atmosphere to two significant figures (e.g. 4.3 m or 43 km).
Use the default collision speed of 20km/s.
b) How much energy is released by the largest airbursts on each planet
(in Megatons)? How often does this happen?
c) What are the smallest craters that can be produced on these planets?
d) Saturn's satellite Titan has an atmosphere about ten times thicker
than Earth's. What sorts of impact craters might you expect to find on
its surface?
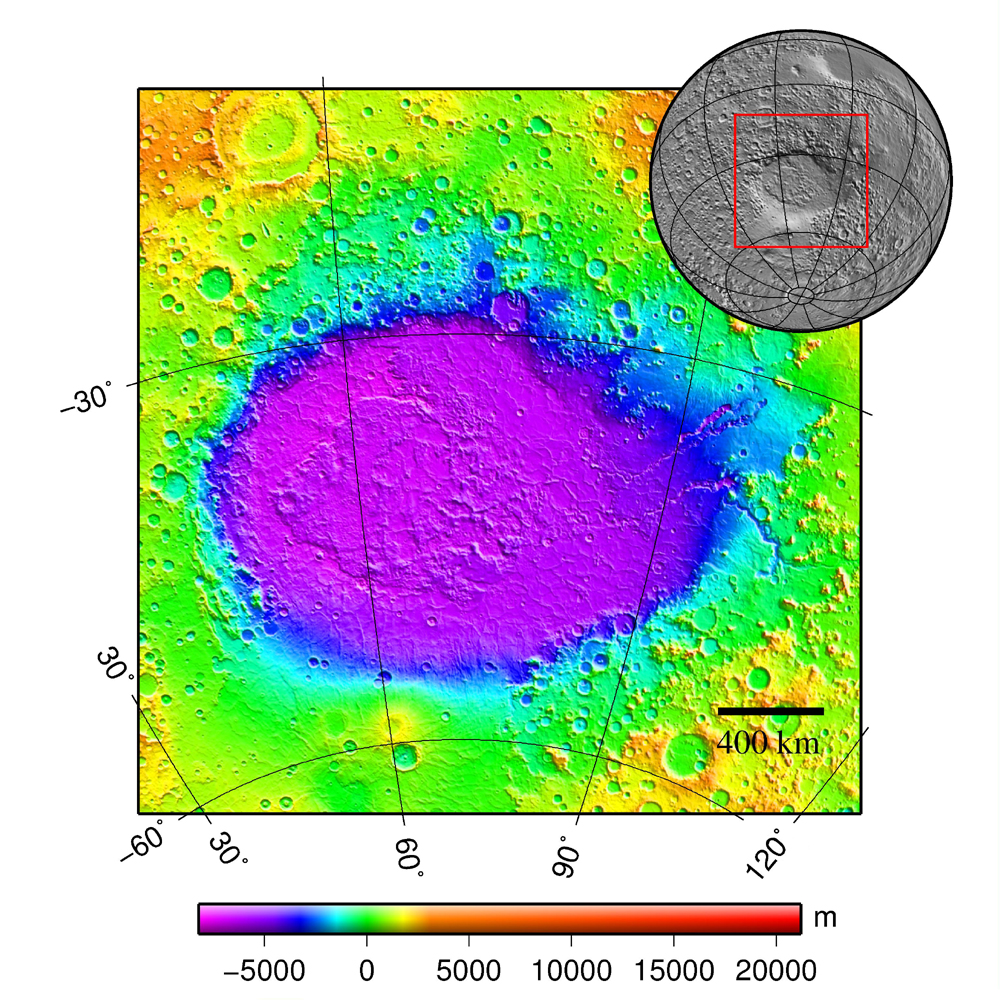 Question 3.
Go to the Solar System
Collisions program.
Question 3.
Go to the Solar System
Collisions program.
a) The Hellas impact basin on Mars is about 1800 km in diameter
and about 6 km deep. Use the Collision Calculator with v=20 km/s to
work out how large the impactor must have been if it was an asteroid
(made of rock) or a comet (made of ice).
b) Use the rocky and
icy impactors from part a) on the Earth and the Moon and compare the crater
diameters and depths to the ones on Mars. Can you guess at the reason
for the differences? Explain your answer.
Question 4. Once more, use the Solar System
Collisions program.
The energy contained in the motion of an
object of mass m moving at speed v is m*v2/2. In this problem, you
will test how well this formula fits the energy released a high speed
impact with Earth.
a) Go back to the table that you created in
HW#1. How much does the impact energy increase for each factor of ten
increase in diameter? Is this consistent with the formula? Why or why
not?
b) Now run several simulations for a 1km object hitting Earth
at different velocities: 15, 30 and 60 km/s. Record the impact
energies and show how well they match the predictions of the
equation. Use ratios as you did in problem 1 of HW#2.
 Back
to the assignments page
Back
to the assignments page
 Question 3.
Go to the Solar System
Collisions program.
Question 3.
Go to the Solar System
Collisions program.
 Back
to the assignments page
Back
to the assignments page